Today's Topic - Decimals Under "Math Magic: Unlocking the Basics" For Students Parents & teachers

 Welcome to our  blog, where we'll explore the fascinating world of decimals and their connection to fractions. In this blog, we'll delve into the basics of decimals, their types, and operations, as well as their relationship to fractions. Whether you're a student, teacher, or simply a curious learner, we invite you to join us on this journey into the world of decimals.

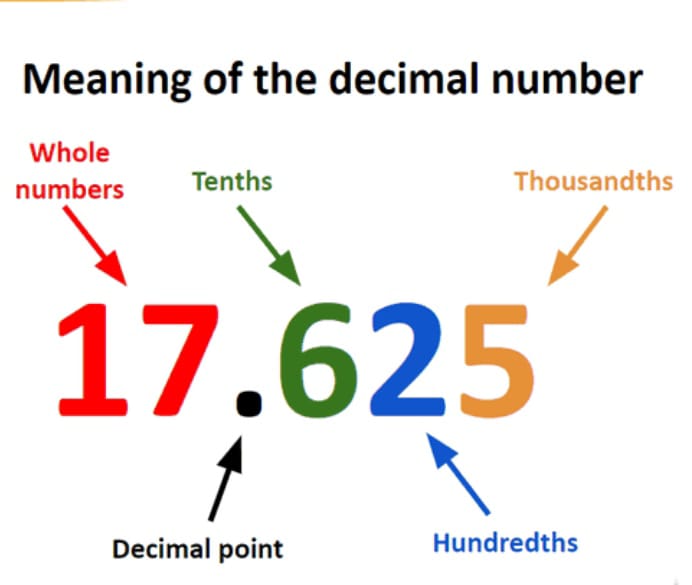
Here's an explanation of decimals made easy through understanding place value:

Before diving into decimals, let's review place value:

- The ones place represents single units (1, 2, 3, etc.).

- The tens place represents groups of 10 (10, 20, 30, etc.).

- The hundreds place represents groups of 100 (100, 200, 300, etc.).

Decimal Place Value-

Decimals extend the concept of place value to include fractions:

- The tenths place represents 1/10 or 0.1.

- The hundredths place represents 1/100 or 0.01.

- The thousandths place represents 1/1000 or 0.001.

Reading Decimals -

To read decimals, combine the whole number part with the fractional part:

- 4.5 = 4 and 5 tenths

- 2.37 = 2 and 37 hundredths

- 1.942 = 1 and 942 thousandths

Writing Decimals -

To write decimals, separate the whole number part from the fractional part with a decimal point:

- 4 and 5 tenths = 4.5

- 2 and 37 hundredths = 2.37

- 1 and 942 thousandths = 1.942

I hope this explanation helps you understand decimals better!

Here are the basics of decimals:

What are Decimals?:

Decimals are a way to represent fractions with a denominator of 10 or a power of 10.

Decimal Notation :

Decimals are written in the following format:

Whole number part + Decimal point + Fractional part

Example: 12.5

Understanding Decimal Places

Each digit after the decimal point represents a power of 10:

- The first digit after the decimal point represents 10^(-1) or 1/10.

- The second digit after the decimal point represents 10^(-2) or 1/100.

- The third digit after the decimal point represents 10^(-3) or 1/1000.

Types of Decimals

There are three types of decimals:

1. Terminating decimals: These are decimals that end or terminate after a certain number of digits. Example: 0.25

2. Recurring decimals: These are decimals that have a repeating pattern of digits. Example: 0.333... (where the 3 repeats indefinitely)

3. Non-terminating and non-recurring decimals: These are decimals that go on indefinitely without repeating. Example: π (pi) = 3.14159....

operations with     decimals:

Adding Decimals

To add decimals, follow these steps:

1. Line up the decimal points.

2. Add zeros to the right of the decimal point if necessary.

3. Add the numbers as you would with whole numbers.

Example: 

   2.5

+ 1.8

  ------

   4.3

Subtracting Decimals:

To subtract decimals, follow these steps:

1. Line up the decimal points.

2. Add zeros to the right of the decimal point if necessary.

3. Subtract the numbers as you would with whole numbers.

  Example:

   4.7 

-  2.3

  ------

   2.4

Multiplying Decimals :

To multiply decimals, follow these steps:

1. Multiply the numbers as you would with whole numbers.

2. Count the total number of decimal places in the factors.

3. Place the decimal point in the product so that it has the same number of decimal places as the total number of decimal places in the factor Example:

   2.4 × 3.5 = ?

   Multiply:   24 × 35 = 840

   Total decimal places: 2 + 1 = 3

   Place decimal point:  8.40

Dividing Decimals :

To divide decimals, follow these steps:   1. Convert the divisor (the number by which you are dividing) to a whole number by moving the decimal point to the right.

2. Move the decimal point in the dividend (the number being divided) the same number of places to the right.

3. Divide the numbers as you would with whole numbers.

Example : 12.6 ÷ 2.4 = ?

  Convert divisor:  2.4 → 24

   Move decimal point in dividend:  12.6 → 126

 Divide:  126 ÷ 24 = 5.25

Rounding Decimal :

Rounding decimals involves approximating a decimal to a certain number of places.

- To round a decimal to the nearest tenth, look at the digit in the hundredths place. If it is 5 or greater, round up. If it is less than 5, round down.

- To round a decimal to the nearest hundredth, look at the digit in the thousandths place. If it is 5 or greater, round up. If it is less than 5, round down.

Example:

 Round 4.27 to the nearest tenth:  4.3

   Round 4.27 to the nearest hundredth:  4.27

Key Concepts

- Decimal point - The point that separates the whole number part from the fractional part.

- Places - The digits after the decimal point, which represent powers of 10.

- Rounding - The process of approximating a decimal to a certain number of places.

Decimals and fractions are closely related. In fact, decimals are a way to represent fractions with a denominator of 10 or a power of 10.

Converting Fractions to Decimals

To convert a fraction to a decimal, divide the numerator by the denominator.

Example:

- 1/2 = 0.5

- 3/4 = 0.75

- 2/5 = 0.4

Converting Decimals to Fractions

To convert a decimal to a fraction, write the decimal as a fraction with a denominator of 10 or a power of 10.

Example:

- 0.5 = 1/2

- 0.75 = 3/4

- 0.4 = 2/5

Equivalent Decimals and Fractions

Some decimals and fractions are equivalent, meaning they represent the same value.

Example:

- 0.5 = 1/2 = 5/10

- 0.75 = 3/4 = 75/100

Real-World Applications

Understanding the relationship between decimals and fractions is important in real-world applications, such as:

- Finance: converting interest rates and investment returns between decimals and fractions.

- Science: representing measurements and data in both decimal and fraction form.

- Cooking: scaling recipes up or down using decimal and fraction equivalents.

I hope this helps clarify the relationship between decimals and fractions!

Thanks for reading our blog on decimals! We hope you learned something new. Our next blog will be on percentages, covering the basics and beyond. See you then!

Note : We will share this information only on Every saturday...

By 

Prof. Swati Pradip Jadhao

WhatsApp Group 👇

https://chat.whatsapp.com/GDjvYdmxJLWFBiOvQajm4Y

Search pradipjadhao on Google for more detail Information.. 

Thank you🙏